12 2.4 Counting Large Numbers of Atoms: The Mole
Learning Objectives
By the end of this section, you will be able to:
- Define the amount unit mole and the related quantity Avogadro’s number
- Explain the relation between mass, moles, and numbers of atoms, and perform calculations deriving these quantities from one another
The identity of an elemental substance is defined not only by the type of atom it contains, but also by the quantity of atoms in the sample. For example, a 1.00 g sample of iron (Fe) contains 1.08 x 1022 atoms of iron. (That is a lot of atoms! If we were to divide those atoms up amongst each of the 7.9 billion people on earth in 2021, each person would have more than a trillion atoms). Because real samples we use in the laboratory will always be composed of very large numbers of atoms, scientists regularly use a unit, the mole, to count the very large quantities.
The mole is an amount unit similar to familiar units like pair, dozen, gross, etc. It provides a specific measure of the number of atoms or molecules in a bulk sample of matter. By definition, a mole is the amount of a substance containing the same number of atoms as the number of atoms in a sample of pure 12C weighing exactly 12 g. One Latin connotation for the word “mole” is “large mass” or “bulk,” which is consistent with its use as the name for this unit. The mole provides a link between an easily measured macroscopic property, bulk mass, and the number of atoms present in a sample.
The number of entities composing a mole has been experimentally determined to be 6.02214179 × 1023, a fundamental constant named Avogadro’s number (NA) or the Avogadro constant in honor of Italian scientist Amedeo Avogadro. For atoms, this constant is properly reported with an explicit unit of “per mole,” a conveniently rounded version being 6.022 × 1023 atoms/mol.
6.022 x 1023 atoms = 1 mole of atoms
Consistent with its definition as an amount unit, 1 mole of any element contains the same number of atoms as 1 mole of any other element. The masses of 1 mole of different elements, however, are different, since the masses of the individual atoms are drastically different. The molar mass of an element is the mass in grams of 1 mole of that substance, a property expressed in units of grams per mole (g/mol) (see Figure 1).
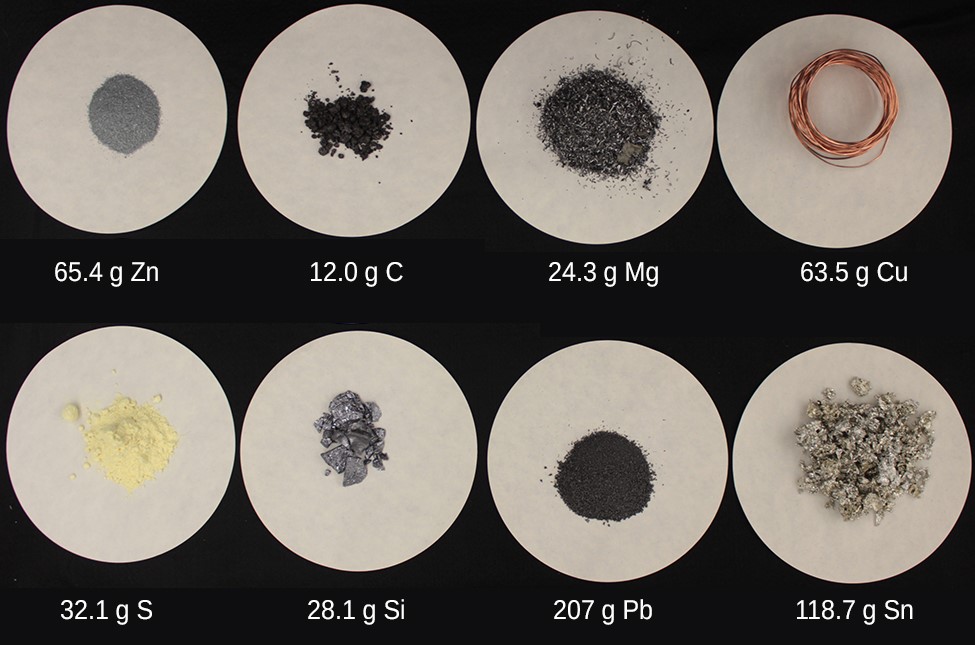
Because the definitions of both the mole and the atomic mass unit are based on the same reference substance, 12C, the molar mass of any substance is numerically equivalent to its atomic or formula weight in amu. Per the amu definition, a single 12C atom weighs 12 amu (its atomic mass is 12 amu). According to the definition of the mole, 12 g of 12C contains 1 mole of 12C atoms (its molar mass is 12 g/mol). This relationship holds for all elements, since their atomic masses are measured relative to that of the amu-reference substance, 12C. We can see this applied to several elements in Table 6. While the numerical values are the same, the different units help us to remember whether we are describing the mass of a single atom or the mass of a very large number of atoms.
Table 6
| Element | Mass of a single atom | Mass of 6.022 x 1023 atoms |
| argon (Ar) | 39.95 amu/atom | 39.95 g/mol |
| iron (Fe) | 55.85 amu/atom | 55.85 g/mol |
| selenium (Se) | 78.96 amu/atom | 78.96 g/mol |

The mole is used in chemistry to represent 6.022 × 1023 of something, but it can be difficult to conceptualize such a large number. Watch this video and then complete the “Think” questions that follow. Explore more about the mole by reviewing the information under “Dig Deeper.”
The relationships atomic mass, the mole, and Avogadro’s number can be applied to compute various quantities that describe the composition of an elemental substances. For example, if we know the mass of an elemental substance, we can determine the number of moles and calculate number of atoms in the sample. Likewise, if we know the number of moles of a substance, we can derive the number of atoms and calculate the substance’s mass.
Example 1
Deriving Moles from Grams for an Element
According to nutritional guidelines from the US Department of Agriculture, the estimated average requirement for dietary potassium is 4.7 g. What is the estimated average requirement of potassium in moles?
Solution
The mass of K is provided, and the corresponding amount of K in moles is requested. Referring to the periodic table, the atomic mass of K is 39.10 amu, and so its molar mass is 39.10 g/mol. The given mass of K (4.7 g) is a bit more than one-tenth the molar mass (39.10 g), so a reasonable “ballpark” estimate of the number of moles would be slightly greater than 0.1 mol.
The molar amount of a substance may be calculated by dividing its mass (g) by its molar mass (g/mol):

The factor-label method supports this mathematical approach since the unit “g” cancels and the answer has units of “mol:”
[latex]4.7 \;\rule[0.5ex]{1.5em}{0.1ex}\hspace{-1.5em}\text{g K} \times \frac{1 \;\text{mol K}}{39.10 \;\rule[0.5ex]{1.5em}{0.1ex}\hspace{-1.5em}\text{g K}} = 0.12 \;\text{mol K}[/latex]
The calculated magnitude (0.12 mol K) is consistent with our ballpark expectation, since it is a bit greater than 0.1 mol.
Check Your Learning
Beryllium is a light metal used to fabricate transparent X-ray windows for medical imaging instruments. How many moles of Be are in a thin-foil window weighing 3.24 g?
Answer:
0.360 mol Be
Example 2
Deriving Grams from Moles for an Element
A liter of air contains 9.2 × 10−4 mol argon. What is the mass of Ar in a liter of air?
Solution
The molar amount of Ar is provided and must be used to derive the corresponding mass in grams. Since the amount of Ar is less than 1 mole, the mass will be less than the mass of 1 mole of Ar, approximately 40 g. The molar amount in question is approximately one-one thousandth (~10−3) of a mole, and so the corresponding mass should be roughly one-one thousandth of the molar mass (~0.04 g):

In this case, logic dictates (and the factor-label method supports) multiplying the provided amount (mol) by the molar mass (g/mol):
[latex]9.2 \times 10^{-4} \;\rule[0.5ex]{3em}{0.1ex}\hspace{-3em}\text{mol Ar} \times \frac{39.95 \;\text{g Ar}}{1 \;\rule[0.5ex]{3em}{0.1ex}\hspace{-3em}\text{mol Ar}} = 0.037 \;\text{g Ar}[/latex]
The result is in agreement with our expectations, around 0.04 g Ar.
Check Your Learning
What is the mass of 2.561 mol of gold?
Answer
504.4 g Ar
Example 3
Deriving Number of Atoms from Mass for an Element
Copper is commonly used to fabricate electrical wire (Figure 7). How many copper atoms are in 5.00 g of copper wire?

Solution
The number of Cu atoms in the wire may be conveniently derived from its mass by a two-step computation: first calculating the molar amount of Cu, and then using Avogadro’s number (NA) to convert this molar amount to number of Cu atoms: Considering that the provided sample mass (5.00 g) is a little less than one-tenth the mass of 1 mole of Cu (~64 g), a reasonable estimate for the number of atoms in the sample would be on the order of one-tenth NA, or approximately 1022 Cu atoms. Carrying out the two-step computation yields:
Considering that the provided sample mass (5.00 g) is a little less than one-tenth the mass of 1 mole of Cu (~64 g), a reasonable estimate for the number of atoms in the sample would be on the order of one-tenth NA, or approximately 1022 Cu atoms. Carrying out the two-step computation yields:
[latex]5.00 \;\rule[0.5ex]{2em}{0.1ex}\hspace{-2em}\text{g Cu} \times \frac{1 \;\rule[0.5ex]{3em}{0.1ex}\hspace{-3em}\text{mol Cu}}{63.55 \;\rule[0.5ex]{2em}{0.1ex}\hspace{-2em}\text{g Cu}} \times \frac{6.022 \times 10^{23} \;\text{atoms Cu}}{1 \;\rule[0.5ex]{3em}{0.1ex}\hspace{-3em}\text{mol Cu}} = 4.74 \times 10^{22} \;\text{atoms Cu}[/latex]
The factor-label method yields the desired cancellation of units, and the computed result is on the order of 1022 as expected.
Check Your Learning
A prospector panning for gold in a river collects 15.00 g of pure gold. How many Au atoms are in this quantity of gold?
Answer
4.586 x 1022 Au atoms
Key Concepts and Summary
Experimental measurements have determined the number of entities composing 1 mole of substance to be 6.022 × 1023, a quantity called Avogadro’s number. The mass in grams of 1 mole of substance is its molar mass. Due to the use of the same reference substance in defining the atomic mass unit and the mole, the formula mass (amu) and molar mass (g/mol) for any substance are numerically equivalent (for example, one iron (Fe) atom weighs approximately 55.85 amu and 1 mole of Fe atoms weighs 55.85 g).
Chemistry End of Chapter Exercises
- What is the atomic mass of boron (B)? What is the molar mass of boron?
- What is the atomic mass of magnesium (Mg)? What is the molar mass of Mg?
- A sample of boron has 1.22 x 1020 atoms in it. Express this quantity in terms of moles of boron.
- A sample of magnesium has 3.25 x 1021 atoms in it. Express this quantity in terms of moles of Mg.
- A sample of calcium contains 0.253 mol of Ca atoms. How many atoms of Ca are in the sample?
- A sample of sulfur contains 0.253 mol of S atoms. How many atoms of S are in the sample?
- You have a 54.3 g sample of lithium. How many moles of Li are in the sample? How many atoms of Li are in the sample?
- You have a 8.73 g sample of Ar. How many moles of Ar are in the sample? How many atoms of Ar are in the sample?
- What is the mass (in g) of a sample of carbon (C) containing 0.576 mol of C?
- What is the mass (in g) of a sample of selenium (Se) containing 0.223 mol of Se?
- What is the mass (in g) of a sample of neon (Ne) containing 7.2 x 1024 atoms of Ne?
- What is the mass (in g) of a sample of aluminum (Al) containing 4.5 x 1024 atoms of Al?
Glossary
- Avogadro’s number (NA)
- 6.022 x 1023 atoms = 1 mole of atoms
- molar mass (g/mol)
- the mass in grams of 1 mole of that substance
- mole
- the amount of a substance containing the same number of atoms as the number of atoms in a sample of pure 12C weighing exactly 12 g
Solutions
Solutions to Chemistry End of Chapter Exercises (even only)
2. What is the atomic mass of magnesium (Mg)? What is the molar mass of Mg?
We can find the numerical value of the atomic mass AND the molar mass on the periodic table. For Mg, the numerical value is 24.30. To differentiate between them we add the appropriate unit values to indicate whether we are talking about the mass of an atom or a mass of a mole of atoms.
atomic mass: 24.30 amu/atom molar mass: 24.30 g/mol
4. A sample of magnesium has 3.25 x 1021 atoms in it. Express this quantity in terms of moles of Mg.
To convert from atoms to moles, we use the relationship that comes from the definition of a mole (6.022 x 1023 atoms = 1 mole of atoms) by using it as a conversion factor. Note that we orient the conversion factor so that the units cancel appropriately. (Insignificant figures are underlined before reporting the final answer).
[latex]3.25 \times 10^{21} \;\rule[0.5ex]{4em}{0.1ex}\hspace{-4em}\text{atoms Mg} \times \frac{1 \;\text{mol Mg}}{6.022 \times 10^{23} \;\rule[0.5ex]{4em}{0.1ex}\hspace{-4em}\text{atoms Mg}} = 0.00539\underline{688} \;\text{mol Mg} = 0.00540 \;\text{mol Mg}[/latex]
6. A sample of sulfur contains 0.253 mol of S atoms. How many atoms of S are in the sample?
To convert from moles to atoms, we use the relationship that comes from the definition of a mole (6.022 x 1023 atoms = 1 mole of atoms) by using it as a conversion factor. Note that we orient the conversion factor so that the units cancel appropriately. (Insignificant figures are underlined before reporting the final answer).
[latex]0.253 \;\rule[0.5ex]{2.5em}{0.1ex}\hspace{-2.5em}\text{mol S} \times \frac{6.022 \times 10^{23} \;\text{atoms S}}{1 \;\rule[0.5ex]{2.5em}{0.1ex}\hspace{-2.5em}\text{mol S}} = 1.52\underline{357} \times 10^{23} \;\text{atoms S} \\ = 1.52 \times 10^{23} \;\text{atoms S}[/latex]
8. You have an 8.73 g sample of Ar. How many moles of Ar are in the sample? How many atoms of Ar are in the sample?
To convert from grams to moles, we use the molar mass of Ar (39.95 g/mol) as a conversion factor. Note that we orient the conversion factor so that the units cancel appropriately. (Insignificant figures are underlined before reporting the final answer).
[latex]8.73 \;\rule[0.5ex]{2em}{0.1ex}\hspace{-2em}\text{g Ar} \times \frac{1 \;\text{mol Ar}}{39.95 \;\rule[0.5ex]{2em}{0.1ex}\hspace{-2em}\text{g Ar}} = 0.218\underline{523} \;\text{mol Ar} = 0.219 \;\text{mol Ar}[/latex]
Now we can convert to atoms as in question 6, above. Notice that we kept and underlined the same insignificant figures to reduce rounding errors.
[latex]0.218\underline{523} \;\rule[0.5ex]{3em}{0.1ex}\hspace{-3em}\text{mol Ar} \times \frac{6.022 \times 10^{23} \;\text{atoms Ar}}{1 \;\rule[0.5ex]{3em}{0.1ex}\hspace{-3em}\text{mol Ar}} = 1.32\underline{595} \times 10^{23} \;\text{atoms Ar} \\ = 1.33 \times 10^{23} \;\text{atoms Ar}[/latex]
10. What is the mass (in g) of a sample of selenium (Se) containing 0.223 mol of Se?
To convert from grams to moles, we use the molar mass of Se (78.96 g/mol) as a conversion factor. Note that we orient the conversion factor so that the units cancel appropriately. (Insignificant figures are underlined before reporting the final answer).
[latex]0.233 \;\rule[0.5ex]{3em}{0.1ex}\hspace{-3em}\text{mol Se} \times \frac{78.96 \;\text{g Se}}{1 \;\rule[0.5ex]{3em}{0.1ex}\hspace{-3em}\text{mol Se}} = 18.3\underline{97} \;\text{g Se} = 18.4 \;\text{g Se}[/latex]
12. What is the mass (in g) of a sample of aluminum (Al) containing 4.5 x 1024 atoms of Al?
For this problem, we need to recognize that there is no relationship that will convert from grams to atoms in a single step. We will need to first convert grams to moles, then moles to atoms. We can do this in two steps (as in question 8), or we can set up the conversion factors together, as shown below. (Insignificant figures are underlined before reporting the final answer).
[latex]4.5 \times 10^{24} \;\rule[0.5ex]{4em}{0.1ex}\hspace{-4em}\text{atoms Al} \times \frac{1 \;\;\rule[0.5ex]{3em}{0.1ex}\hspace{-3em}\text{mol Al}}{6.022 \times 10^{23} \;\rule[0.5ex]{4em}{0.1ex}\hspace{-4em}\text{atoms Al}} \times \frac{26.98 \;\text{g Al}}{1 \;\rule[0.5ex]{3em}{0.1ex}\hspace{-3em}\text{mol Al}}= 20\underline{16.107} \;\text{g Al} = 2.0 \times 10^{3} \;\text{g Al}[/latex]

